 |
 |
|
|
Input Description: A set \(S\) of \(n\) points in \(d\)-dimensional space.
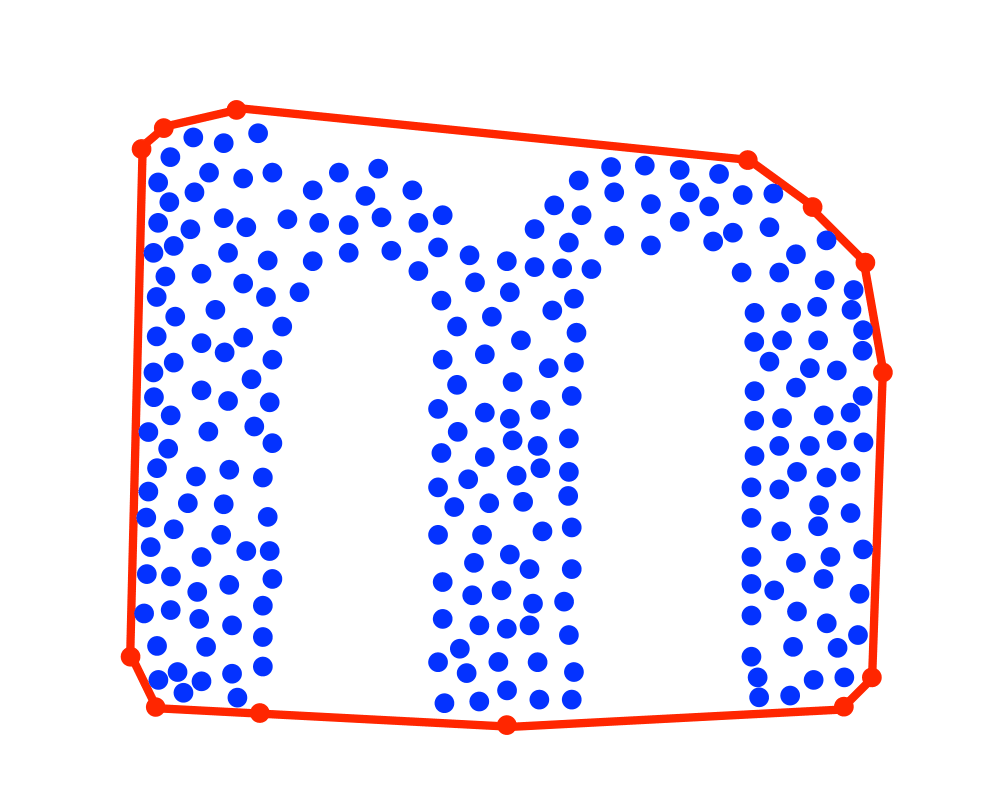
Problem: Find the smallest convex polygon containing all the points of \(S\).
Excerpt from The Algorithm Design Manual: Finding the convex hull of a set of points is the most elementary interesting problem in computational geometry, just as minimum spanning tree is the most elementary interesting problem in graph algorithms. It arises because the hull quickly captures a rough idea of the shape or extent of a data set.
Convex hull also serves as a first preprocessing step to many, if not most, geometric algorithms. For example, consider the problem of finding the diameter of a set of points, which is the pair of points a maximum distance apart. The diameter will always be the distance between two points on the convex hull. The O(n \lg n). algorithm for computing diameter proceeds by first constructing the convex hull, then for each hull vertex finding which other hull vertex is farthest away from it. This so-called ``rotating-calipers'' method can be used to move efficiently from one hull vertex to another.
  Simplifying Polygons |
  Sorting |
  Traveling Salesman Problem |
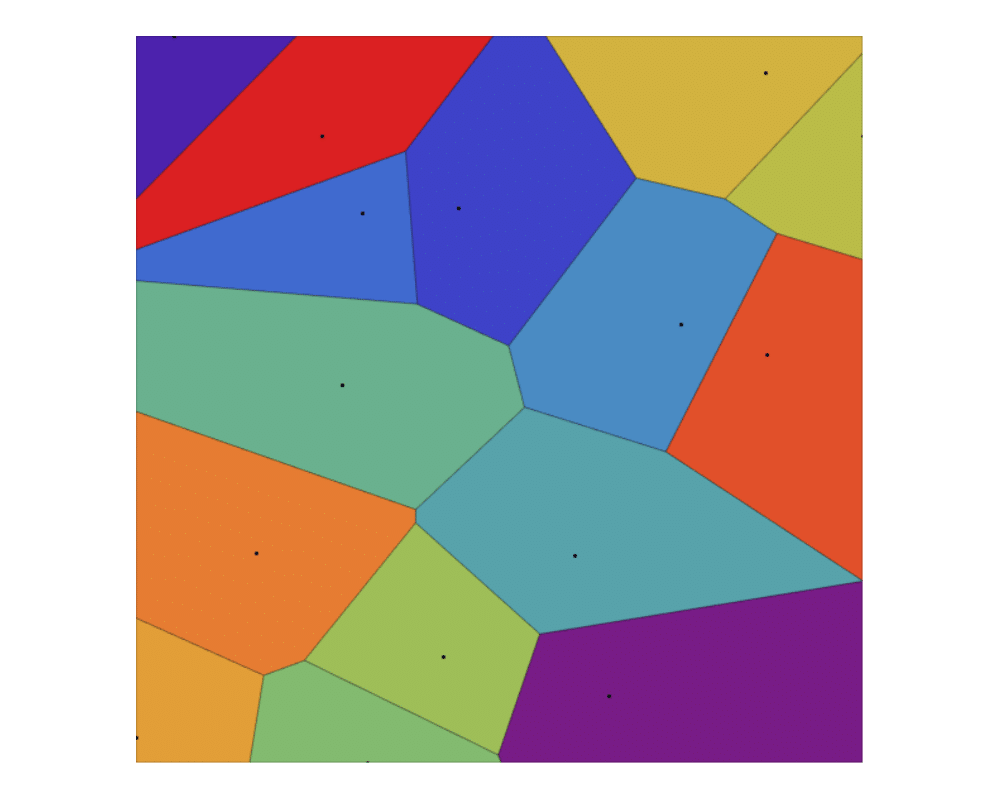  Voronoi Diagrams |
As an Amazon affiliate, I earn from qualifying purchases if you buy from links on this website.
Computational Geometry : Algorithms and Applications by Mark De Berg, Marc Van Kreveld, Mark Overmars, and O. Schwartskopf
Computational Geometry in C by Joseph O'Rourke
Algorithms from P to NP, Vol. I: Design and Efficiency by B. Moret and H. Shapiro
Introduction to Algorithms by T. Cormen and C. Leiserson and R. Rivest and C. Stein